The relevant equation is given by
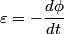 where 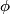 is the magnetic flux through the loop as a function of time, and is the magnetic flux through the loop as a function of time, and  is the motional emf induced in the loop. %This is not an application of Faraday's Law, as discussed on page 303 of Griffiths EM, although the same formula holds for applications of Faraday's Law as well. is the motional emf induced in the loop. %This is not an application of Faraday's Law, as discussed on page 303 of Griffiths EM, although the same formula holds for applications of Faraday's Law as well. The sign of the induced emf and the direction of the current can be obtained in two ways. One way is by using Lenz's Law%, which DOES apply to motional emfs as stated in footnote 7 on page 303 of Griffiths EM. The other way is by recalling that  and 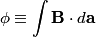 where 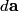 is determined by the sense in which the loop in equation (1) is traversed and by an application of the right-hand corkscrew rule. is determined by the sense in which the loop in equation (1) is traversed and by an application of the right-hand corkscrew rule. Using either way, we find that the current induced in the loop in this problem flows clockwise. Therefore, ``positive charges are moving down on the right part of the loop" and ``positive charges are moving up on the left part of the loop." The magnetic field is directed into where the loop is. Therefore, by the Lorentz Force law, the force on the right part of the current loop is directed to the right and the force on the left part of the current loop is directed to the left. Therefore, answer (E) is correct. |